The leading term of a polynomial is the term that contains the variable raised to the highest power. For example, in the polynomial \(3x^2-5x+2\), the leading term is \(3x^2\).
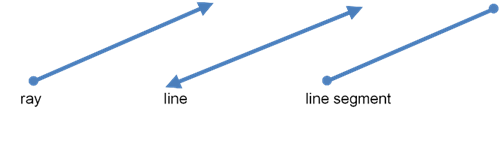
In geometry, a line extends infinitely in both directions.
A line is different from a ray (which extends from a point toward infinity) and a line segment (which extends between two points). Lines are depicted with arrow heads on both ends to distinguish them from rays and line segments.
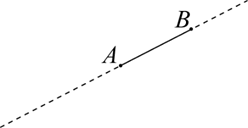
If A and B are two points on a line, the part of the line between and including A and B is called a line segment or interval.
The distance AB is a measure of the length of AB.
A linear equation is an equation involving just linear terms, that is, no variables are raised to a power greater than one. The general form of a linear equation in one variable is \(ax+b=0\), where a and b cannot both be 0. The solution of a linear equation in general form is \(x=\frac{-b}a\)
A linear equation with two variables takes the general form \(ax+by+c=0\), where a and b cannot both be 0. If a≠0, then the point where the line intersects the x-axis (the x-intercept) is \(\frac ca\).
If b≠0, then the point where the line intersects the y-axis (the y-intercept) is \(\frac cb\).
Two-variable, or two-dimensional linear equations also come in the form \(y=mx+b\). The constant m indicates the gradient or slope of a line, while b represents the y-intercept.
In Measurement and Geometry, the relative position of an object is referred to as its location. It can be expressed in terms of a description (such as ‘next to’, ‘behind’, ‘on top of’), a grid reference (such as ‘A5’), or a coordinate (such as ‘(-2,7)’).
In Statistics and Probability, a measure of location is a single number that can be used to indicate a central or ‘typical value’ within a set of data. The most commonly used measures of location are the mean and the median although the mode is also sometimes used for this purpose.
The logarithm of a positive number x is the power to which a given number b, called the base, must be raised in order to produce the number x. The logarithm of x, to the base b is denoted by \(\;\log_bx\).
Algebraically, the statements \(\log_bx=y\) and \(b^y=x\) are equivalent in the sense that both statements express the identical relationship between x, y and b. For example, \(\log_{10}\;100=2\) because \(10^2=100,\), and \(\log_2\left(\frac1{32}\right)=-5\) because \(2^{-5}=\frac1{32}\).